Surface Area of a Cone Calculator

In the article below you will read about the Surface Area of a Cone Calculator. You will learn what it is, its formula, how to calculate it, and what is this calculator used for. Also, you will learn all about the surface area of a right cone. If you want to know more about it keep reading, and at the end, there will be some answered questions to what people usually ask when they hear about this calculator.

As you are reading through this article keep in mind that some people refer to surface area of a cone as surface area of a right circular cone.

Some of the similar posts to this one are Area Calculator, Golden ratio and almost the same one, but for volume, Cone Volume Calculator. Also, try out this our Volume Calculator and Volume Conversion as well. There is also our Surface Area of a Rectangular Prism Calculator.

What is the Surface Area of a Cone?

First thing, you need to know what a cone is. With this in mind, the cone is a solid that is generated when the right triangle circles around one of its “legs” or vertices. That is why it is so similar to a pyramid, but its base is a circle instead of a cube. Now, what is the surface area of a cone? The surface area is the sum of the base area and the area of the lateral (side) surface.

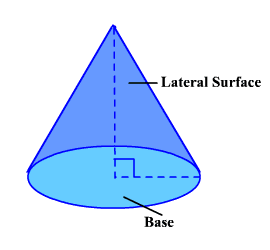
This image shows us exactly what the lateral surface is and what is the base.

What is a Right Cone?

To know what a right cone is, you must know that there are 2 types of cones:

• right circular cone
• oblique cone

The axis of the right circular cone is perpendicular to its base and the axis of the oblique cone is tilted, so it’s not perpendicular to its base.

The radius of this cone is the distance between the center of the base and any point on the circumference of the base, while the height is the distance between the center of the base and the apex of a cone.

Lastly, the slant height is the distance between the apex of a cone and any point on the circumference of its base.

Surface Area of a Right Cone Formula

To explain this, you must know that there are 2 types of surface area that need to be calculated:

• Curved surface area or CSA
• Total surface area or TSA

Curved surface area is the area of the curved surface of a cone, and the total surface area is the area of the curved surface, including the area of its base:

CSA = \pi \cdot r \cdot s

CSA = \pi \cdot r \cdot \sqrt {r^2+h^2}

Where:

• r is the radius of the base
• h is the height
• s is the slant height

So, what is the formula for the surface area of a cone? The equation for this surface is the same as the formula for total surface area, like I have said that surface area is a sum of the base area and lateral surface area. In short:

TSA = \pi \cdot r^2 + \pi \cdot r \cdot s

TSA = \pi \cdot r^2 + \pi \cdot r \cdot \sqrt {r^2+h^2}

As for units, you should be using all units of length. For example: meters, inches, centimeters, etc.

What type of cone is this calculator used for?

This calculator is used to calculate the surface area of just one type of cone, which is the right cone. Therefore, all of the formulas in this calculator can be only used for this single type of cone.

Surface Area of a Cone Calculator – How to Use?

Next important thing about this calculator is how to use it. So, let’s go through the steps on how to use this calculator:

1. First step of what we need to do, is to enter the given radius of a circular base. Don’t forget that the radius is half of the circle’s diameter and a diameter of a circle is line that goes across the circle through its center. If you don’t have a radius, as an alternative, you can use the circumference of the base.
2. Second step is to enter the given height, or the slant height, where the height must always be greater than zero.
3. Lastly, you will get the result in the units of area that have been entered beforehand in the first and second steps. Be careful with those units, because you should be using only the same ones, or your calculations will be wrong.

Surface Area of a Cone Calculator – Example

Example 1:

Find the curved surface area of a cone with the radius of 4 cm and the slant height of 5 cm.

Solution:

CSA = \pi \cdot 4 \cdot 5 = 20 \cdot \pi \approx62,82 \; cm^2

For example, with given radius of 4 cm and slant height of 5 cm, our wanted surface is 62.82 square centimeters. You can convert between different square units with this Area Conversion Calculator.

Example 2:

Find the total surface area of a cone given that radius is 6 inches and the slant height is 10 inches.

Solution:

TSA = \pi \cdot 6^2 + \pi \cdot 6 \cdot 10 = 60 \cdot \pi + 36 \cdot \pi \approx 301,44 \; in^2

Check these results with our calculator, and choose different units as the output value, such as square meters.

FAQ