Fraction to Percent calculator is used to quickly and easily convert a fractional expression to a percent of it. It uses the standard formula for the conversion of percent, and it supports both directions: fraction-to-percent and percent-to-fraction. The use of Fraction to Percent calculator is not only present in math or other similar sciences. Moreover, people use it regularly in real-life scenarios, even though you may not be quite aware of it. Thus, it would be wrong to say that those calculations are only of use to scientists.
If you need more math-related calculators besides the Fraction to Percent Calculator, check out this category.
How to convert fraction to percent
Before we show the process of how to convert fraction value into percent value, it is mandatory first to give their definitions.
A fraction is an expression when we divide two numbers, and we do not get a prime number from the equation. Then, we express that number as a fraction of its numerator and denominator. There is our Least Common Denominator Calculator, for you to handle better with adding, subtracting and other operations.
A percent is an expression when we write a particular fraction as a number out of 100.
Fraction to Percent
We get the percent value from a fraction by dividing its numerator by the denominator. Then, when we get the decimal number from that operation, we need to multiply the result by 100. The final number we get is expressed in percents.
This is the simplest method to do the conversion. In addition, you can calculate both proper, improper and mixed fractions using this method.
We use percentages every single day for multi-purposes and knowing how to calculate them from a given fraction is very important.
Proper vs improper fractions
A proper fraction is a fraction in which the numerator is smaller than the denominator.
Opposed to the proper, improper is a fraction in which the numerator is an equal or larger number than the denominator.
Fraction to percentage formula
Okay, we saw how fraction-to-percent works, but what is the formula for it. After all, is there any formula defined for converting fractional numbers to percentage numbers in math? The answer is yes, so let’s take a look at the following formula that we use:
P = n \div d \times 100n – top number of the fraction
d – bottom number of the fraction
% – percent sign
So, what are the procedure or steps that we take when we use this percentage formula:
First step: Set up the given fraction and divide numerator by denominator
top number / bottom number= decimal number
Second step: Take the decimal and multiply it by 100
decimal number x 100 = percent
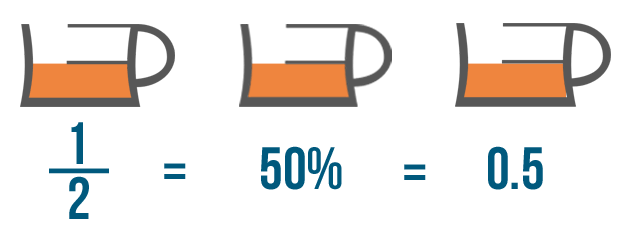
Fraction to percent conversion table
Here is a list of some most common fractions, their equivalent percent value and decimal value. You can use it as a reference for simple fraction calculations so that you don’t need to calculate it manually.
| Fraction | Percent | Decimals |
| 1/12 | 8.33% | 0.083 |
| 1/10 | 10% | 0.1 |
| 1/8 | 12.5% | 0.125 |
| 1/6 | 16.6% | 0.166 |
| 1/5 | 20% | 0.2 |
| 1/4 | 25% | 0.25 |
| 1/3 | 33% | 0.33 |
| 3/8 | 37.5% | 0.375 |
| 2/5 | 40% | 0.4 |
| 1/2 | 50% | 0.5 |
| 3/5 | 60% | 0.6 |
| 5/8 | 62.5% | 0.625 |
| 2/3 | 66.67% | 0.668 |
| 3/4 | 75% | 0.75 |
| 4/5 | 80% | 0.8 |
| 7/8 | 87.5% | 0.875 |
| 1 | 100% | 1.0 |
Converting Fractions to Percent: An example
From the previous example in the formula section, we only gave a brief example of how to do fraction-to-percent conversion. However, in this section, I will go through a real-life example and show you how to use our Fraction to Percent calculator. Not all values are available in the reference percent conversion table, so it’s quicker and easier to use the percent converter than the above table.
Let’s imagine this scenario. There was a survey on who the most popular football player is in the world, at the moment. People can vote for Messi or Ronaldo. You get the result of the survey in a fractional form, three-fifths (fraction bar 3/5) for Messi and two-fifths for Ronaldo. Now, how do we convert those values into percents?
Let’s go step by step:
1) Find how many voters chose Messi in percents
3 \div 5 \times 100 = 60% = decimal equivalent for this is 0.6
2) Find how many voters chose Ronaldo in percents
2 \div 5 \times 100 = 40% = decimal equivalent for this is 0.4
If you want to check if the calculation was correct, you only need to add these two values, and if they equal 100, it means your calculation was correct.
Converting Percent to Fractions: An example
Hopefully, you figured out how the conversion from a fraction to a percent works, but can we do it in the opposite way? The answer is yes, again. I will show you the steps you need to follow in order to convert percents into fractions.
Example:
Value in percents = P
Value in decimals = D
Fraction = F
P \div 100 = D = D \times 1000 \div 1000 = (D \times 1000) \div 1000 F = (D \times 1000) \div 1000As you can see, this is a formula used for converting percent to a fraction. Let’s define some values and use them in the formula.
Scenario: You bought a pizza and ate 55% of it. How can we know how much of that is in a fraction?
55% of pizza converted to decimals is 0.55
We take the decimal, multiply and divide it by 100 to remove two decimal points, which follows 55 / 100.
Then, in order to simplify the fraction, we need to find the greatest common factor of 55 and 100, which is 5.
Once we find the factor, divide the fraction by that number:
55 \div 5 and 100 \div 5
Finally, we get the fraction equivalent of 55%, which is 11 / 20.
Example: Convert 75% to a fraction
The example above showed how it is done using a formula and calculating everything yourself. But, importantly, you would never do it manually because there are calculators for that. Thus, in this example, I will use our Fraction to Percent calculator that will quickly give me a fraction of the percent that I give to it.
75% equivalents to 3/4 in a fractional form
Note: If you calculate from percent (greater than 100%) to fraction, it will return an improper fraction.
FAQ
How do you write 20% as a fraction?
20% of something converted to decimals is 0.2. Then, we multiply and divide it by 10 to remove one decimal point, which follows 2 / 10. In order to simplify the fraction, we need to find the greatest common factor of 2 and 10, which is 2. Once we find the factor, divide the fraction by that number:
2 \div 2 and 10 \div 2
Finally, we get the fraction equivalent of 20%, which is 1/5.
What is 15% turn into a fraction?
Let’s use the same percentage method as in the previous example.
Decimals equivalent of 15% is 0.15. As it has two decimals, we need to multiply it by 100 to get rid of them. 0.15 turns into 15, so now divide it by 100, which looks as follows: 15/100. Let’s find the greatest common factor for the following numbers:
15 \div 5 and 100 \div 5
Finally, we get the fraction equivalent of 15%, which is 3/20.
