Here you can read about our new cross-multiplication calculator. We will present the expressions used in this calculation, called proportions, and cross-multiplying fractions can easily calculate that. The calculator will help you calculate the unknown X by entering the numerator and denominator values. Whichever given value is unknown, it is possible to obtain a final solution. But before that, we need to get acquainted with the basic information available to you below.
What is cross multiplication?
Before getting acquainted with the concept of cross-multiplication, it is necessary to say some basics about parts of fractions. This method uses fractions that make numbers written in the form a / b where a and b number, with b being any number except zero. The number at the top of the fraction is called the numerator, while the number below is the denominator. Both numbers are separated by a fractional or slash. If rational expressions are used, they have the same form: A and b are algebraic expressions instead of integers. Math as science defines cross-multiplication as a situation when an unknown variable in an equation is determined by the cross-multiplication of fractions. You can also use this method to compare fractions. An unknown variable is a place that denotes a specific number or quantity, the value of which is obtained by solving an equation. Cross multiplication is also useful when trying to solve a ratio.
Cross multiplication method
The method used to calculate the unknown value is called the cross-multiplication method. In this situation, the value of the numerator of the first fraction is multiplied by the value of the second fraction’s denominator. The process consists of essential operational multiplication math functions, where:
a\cdot b = c\cdot d
The crossing needs to be done to compare these multiplied values later, especially in the case of more significant fractions. It is also common to use cross-multiplication in examples of equations with one unknown expressed in fractions. Then we can come across fractions that contain negative numbers or decimals, either in denominator or numerator. By equalizing the given values, the value of the unknown variable is determined. When it comes to solving equations in two variables, the simplest way is by cross-multiplication.
How to do cross multiplication?
If you wonder how to do the cross-multiplication procedure, we will now explain the process in detail in just a few steps. The first step involves multiplying the numerator of the left fraction by the denominator of the suitable fraction. In this case, you have an equation that looks like this:
\frac{4}{x} = \frac{10}{15}Then you need to multiply 4 by 15, equal to 60. In the second step, you need to multiply the correct fraction’s numerator and the left fraction’s denominator. Then multiply the unknown x by the number 10, equal to 10x. The direction of multiplication is not essential as long as multiplication takes place diagonally. You need to equalize these two obtained products, which will look like this:
4\cdot 15 = x\cdot 10From this equation, we get that the unknown x has the value 6. With this calculation procedure, our calculator, which we are talking about today, works. The calculator contains four blank fields that correspond to the letters of the numerator and denominator of fractions. The instructions given for CalCon’s cross-multiplication calculator involve entering three values. The fourth and unknown variable will be calculated automatically.
Cross multiplication formula
In practice, this math form of calculation formula is most often used:
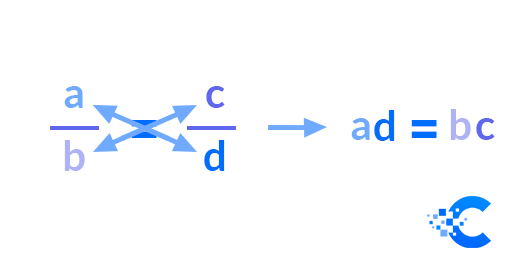
If we are talking about equations with unknown variables, then it looks like this:
Cross multiplication – an example
The cross-multiplication method can be performed in just three steps. In the following example, we can see what this procedure looks like:
Let us say that we are given two fractions:
\frac{x}{7} = \frac{18}{30}and that we need to calculate the unknown value of x.
We can solve the task in two ways:
Method I:
\frac{x}{7} = \frac{18}{30} 30\cdot x = 7\cdot 18 30\cdot x = 126 x = \frac{126}{30} = 4.2
Method II:
In both cases, we obtained the exact value of 4.2. The same procedure would be done if the unknown x had the denominator position.
This calculator is great for children learning multiplication, adults who need to do multiplication without paper and pencil, and those who need to calculate the cost of their grocery list.
